Question

Find the value of annuity if the periodic deposit is $1500 at 8% compounded semiannually for 22 years

Answer 1

The value of annuity is
`P_v = \$ 32058`

Explanation:

From the question we are told that

The periodic payment is
`P = \$ 1500`

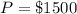
The interest rate is
`r = 8\% = 0.08`

Frequency at which it occurs in a year is n = 2 (semi-annually )

The number of years is
`t = 22 \ years`

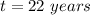
The value of the annuity is mathematically represented as

`P_v = P * [1 - (1 + (r)/(n) )^(-t * n) ] * [((1 + (r)/(n) ))/( (r)/(n) ) ]`(reference EDUCBA website)

substituting values

`P_v = 1500 * [1 - (1 + (0.08)/(2) )^(-22 * 2) ] * [((1 + (0.08)/(2) ))/( (0.08)/(2) ) ]`

`P_v = 1500 * [1 - (1.04 )^(-44) ] * [((1.04 ))/(0.04) ]`

`P_v = 1500 * [1 - 0.178 ] * [((1.04 ))/(0.04) ]`

`P_v = \$ 32058`