Question

What is the focus of the parabola? y=−1/4x2−x+3

Answer 1

Answer: Focus = (-2, 3)

Explanation:

`y=-(1)/(4)x^2-x+3\\\\\rightarrow a=-(1)/(4),\ b=-1`

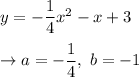
First let's find the vertex. We do that by finding the Axis-Of-Symmetry:

`AOS: x=(-b)/(2a)\quad =(-(-1))/(2((-1)/(4)))=(1)/(-(1)/(2))=-2`

Then finding the maximum by inputting x = -2 into the given equation:

`y=-(1)/(4)(-2)^2-(-2)+3\\\\y=-1+2+3\\\\y=4`

The vertex is: (-2, 4)

Now let's find p, which is the distance from the vertex to the focus:

`a=(1)/(4p)\\\\\\-(1)/(4)=(1)/(4p)\\\\\\p=-1`

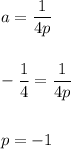
The vertex is (-2, 4) and p = -1

The focus is (-2, 4 + p) = (-2, 4 - 1) = (-2, 3)