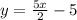Question

Consider this system of equations. Which equation represents the first equation written in slope-intercept form? 5 x minus 2 y = 10. Y = one-fourth x + 1.

Answer 1

`y = (5x)/(2) - 5`

Explanation:

Given the equation 5x - 2y = 10, to write the equation in slope-intercept form, we need to write it in the standard format y = mx+c where m is the slope/gradient and c is the intercept.

From the equation given 5x - 2y = 10, we will make y the subject of the formula as shown;

`5x - 2y = 10\\\\subtract \ 5x \ from \ both \ sides\\\\5x - 2y - 5x = 10 - 5x\\\\-2y = 10-5x\\\\Dividing \ both \ sides\ by \ -2;\\\\(-2y)/(-2) = (10-5x)/(-2)\\ \\`

`y = (10)/(-2) - (5x)/(-2) \\\\y = -5 + (5x)/(2)\\\\y = (5x)/(2) - 5`

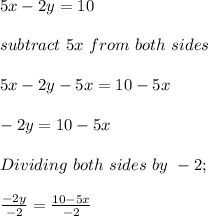
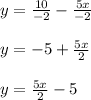
Hence the equation that represents the first equation written in slope-intercept form is
`y = (5x)/(2) - 5`