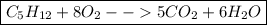Question

May I know how to balance this

Answer 1

C₅H₁₂ + 8O₂ → 5CO₂ + 6H₂O

Step-by-step explanation:

Let's start by counting how many molecules we have on each side.

Reactants

C - 5

H - 12

O - 2

Products

C - 1

O - 3

H - 2

We have to balance both sides. Since we have 5 carbon atoms on the reactants side and 1 carbon atom on the products side, we'll start with that. Write 5 as the coefficient for CO₂ to balance the carbons.

C₅H₁₂ + O₂ → 5CO₂ + H₂O

Now that the carbons are balanced, let's look at the hydrogens. There's 12 on the reactants side and 2 on the products side. To balance the hydrogens, we have to write 6 as the coefficient for H₂O.

C₅H₁₂ + O₂ → 5CO₂ + 6H₂O

Now the hydrogens are balanced. All that's left to do is balance the oxygens. Let's start by counting how many atoms we have on each side.

Reactants

C - 5

H - 12

O - 2

Products

C - 5

H - 12

O - 16

So, to balance the number of atoms on both sides, write 8 as the coefficient for O₂.

C₅H₁₂ + 8O₂ → 5CO₂ + 6H₂O

Lastly, let's check to make sure everything is balanced.

Reactants

C - 5

H - 12

O - 16

Products

C - 5

O - 16

H - 12

Both sides are balanced. Therefore, the balanced chemical equation is: C₅H₁₂ + 8O₂ → 5CO₂ + 6H₂O.

Hope that helps.

Answer 2

`\boxed{C_(5)H_(12) + 8O_(2) -->5CO_(2) + 6H_(2)O}`

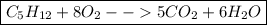
Step-by-step explanation:

Part 1: Count the number of atoms on each side per element

To start, count the number of atoms per element on both sides of the equation.

Left Side

C - 5 atoms

H - 12 atoms

O - 2 atoms

Right Side

C - 1 atom

H - 2 atoms

O - 3 atoms

Part 2: Balance carbon atoms

Now, with this information, you can begin finding out how to properly balance both sides of the equation.

You need 5 carbon atoms on the left, so place a coefficient of 5 in front of the
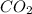`CO_(2)` product. This will balance the carbons. Do not place a coefficient in front of the
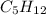`C_(5)H_(12)` reactant - none are necessary!

Then, you can update your counts for your atoms.

Left Side

C - 5 atoms

H - 12 atoms

O - 2 atoms

Right Side

C - 5 atoms

H - 2 atoms

O - 11 atoms

The updated equation will look like this:

`\boxed{C_(5)H_(12)+O_(2) --> 5CO_(2) + H_(2)O}`

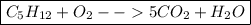
Part 3: Balance hydrogen atoms

Now, balance the hydrogen atoms. Place a coefficient of 6 in front of the
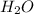`H_(2)O` product. This will balance the hydrogen atoms.

Once again, update the atom counts:

Left Side

C - 5 atoms

H - 12 atoms

O - 2 atoms

Right Side

C - 5 atoms

H - 12 atoms

O - 16 atoms

The updated equation will look like this:

`\boxed{C_(5)H_(12) +O_(2)--> 5CO_(2) +6H_(2)O}`

Part 4: Balance oxygen atoms

To fully balance the equation, place a coefficient of 8 in front of the
`O_(2)` reactant. This will equalize the amount of oxygen atoms on both sides of the equation.

The updated equation will look like this:

`\boxed{C_(5)H_(12) + 8O_(2) --> 5CO_(2) + 6H_(2)O}`

Part 5: Check the equation and atom counts

Now, update the atom counts one last time to make sure they are equal.

Left Side

C - 5 atoms

H - 12 atoms

O - 16 atoms

Right Side

C - 5 atoms

H - 12 atoms

O - 6 atoms

They are equal, so you may proceed.

Part 6: Check if reductions are necessary

If the equations coefficients can all be divided by a common divisor (i.e., 3), follow up with that step. However, any equation that has a reactant or product without a coefficient, skip this step entirely.

This equation falls into that category, so you may proceed past it.

Your final equation will look like this:
`\boxed{C_(5)H_(12) + 8O_(2) -->5CO_(2) + 6H_(2)O}`