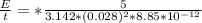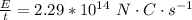Question

A parallel-plate capacitor in air has circular plates of radius 2.8 cm separated by 1.1 mm. Charge is flowing onto the upper plate and off the lower plate at a rate of 5 A. Find the time rate of change of the electric field between the plates.

Answer 1

The time rate of change of the electric field between the plates is
`(E )/(t) = 2.29 *10^(14) \ N \cdot C \cdot s^(-1)`

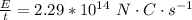
Step-by-step explanation:

From the question we are told that

The radius is
`r = 2.8 \ cm = 0.028 \ m`

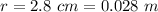
The distance of separation is
`d = 1.1 \ mm = 0.0011 \ m`

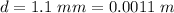
The current is
`I = 5 \ A`

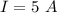
Generally the electric field generated is mathematically represented as

`E = (q )/( \pi * r^2 \epsilon_o )`

Where
`\epsilon_o` is the permitivity of free space with a value

`\epsilon_o = 8.85*10^(-12 )\ m^(-3) \cdot kg^(-1)\cdot s^4 \cdot A^2`

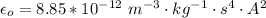
So the time rate of change of the electric field between the plates is mathematically represented as

`(E )/(t) = (q)/(t) * (1 )/( \pi * r^2 \epsilon_o )`

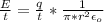
But
`(q)/(t ) = I`

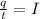
So

`(E )/(t) = * (I )/( \pi * r^2 \epsilon_o )`

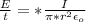
substituting values

`(E )/(t) = * (5 )/(3.142 * (0.028)^2 * 8.85 *10^(-12) )`

`(E )/(t) = 2.29 *10^(14) \ N \cdot C \cdot s^(-1)`