Answer:
B.
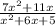
Explanation:
The sum is worked as shown below:
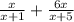
Use the common denominator to divide each denominator, then use the result to multiply the numerator, you'd have the following:
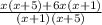
Use the distributive property of multiplication to solve

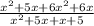
Pair like terms
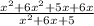
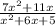
The answer is B.