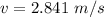Question

A mass M = 4 kg attached to a string of length L = 2.0 m swings in a horizontal circle with a speed V. The string maintains a constant angle \theta\:=\:θ = 35.4 degrees with the vertical line through the pivot point as it swings. What is the speed V required to make this motion possible?

Answer 1

The velocity is
`v = 2.84 1 \ m/s`

Step-by-step explanation:

The diagram showing this set up is shown on the first uploaded image (reference Physics website )

From the question we are told that

The mass is m = 4 kg

The length of the string is
`L = 2.0 \ m`

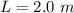
The constant angle is
`\theta = 35.4 ^o`

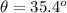
Generally the vertical forces acting on the mass to keep it at equilibrium vertically is mathematically represented as

`Tcos (\theta ) - mg = 0`

=>
`mg = Tcos (\theta )`

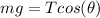
Now let the force acting on mass horizontally be k so from SOHCAHTOA rule

`sin (\theta ) = (k )/(T)`

=>
`k = T sin \theta`

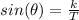
Now this k is also equivalent to the centripetal force acting on the mass which is mathematically represented as

`F_v = (m v^2)/(r)`

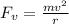
So

`k = F_v`

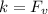
Which

=>
`T sin \theta= ( m v^2)/( r )`

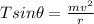
So

`(Tsin (\theta ))/(Tcos (\theta )) = (mg)/( (mv^2)/(r) )`

=>
`Tan (\theta ) = (v^2)/( r * g )`

=>
`v = √(r * g * tan (\theta ))`

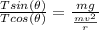
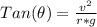
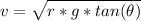
Now the radius is evaluated using SOHCAHTOA rule as

`sin (\theta) = ( r)/(L)`

=>
`r = L sin (\theta)`

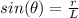
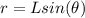
substituting values

`r = 2 sin ( 35.4 )`

`r = 1.1586 \ m`

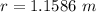
So

`v = √(1.1586* 9.8 * tan (35.4 ))`

`v = 2.84 1 \ m/s`