Answer:
1) AD=BC(corresponding parts of congruent triangles)
2)The value of x and y are 65 ° and 77.5° respectively
Explanation:
1)
Given : AD||BC
AC bisects BD
So, AE=EC and BE=ED
We need to prove AD = BC
In ΔAED and ΔBEC
AE=EC (Given)
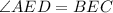 ( Vertically opposite angles)
( Vertically opposite angles)
BE=ED (Given)
So, ΔAED ≅ ΔBEC (By SAS)
So, AD=BC(corresponding parts of congruent triangles)
Hence Proved
2)
Refer the attached figure

In ΔDBC
BC=DC (Given)
So,
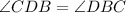 (Opposite angles of equal sides are equal)
(Opposite angles of equal sides are equal)
So,

So,
 (Angle sum property)
(Angle sum property)
x+x+50=180
2x+50=180
2x=130
x=65
So,

Now,
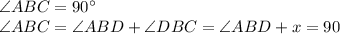
So,
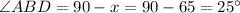
In ΔABD
AB = BD (Given)
So,
 (Opposite angles of equal sides are equal)
(Opposite angles of equal sides are equal)
So,
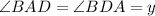
So,
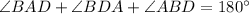 (Angle Sum property)
(Angle Sum property)
y+y+25=180
2y=180-25
2y=155
y=77.5
So, The value of x and y are 65 ° and 77.5° respectively