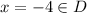Answer:
c. x = -4 or x = 9
Explanation:
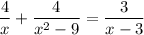
Domain:
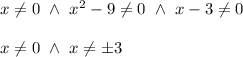
solution:

use (a - b)(a + b) = a² - b²
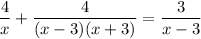
multiply both sides by (x - 3) ≠ 0
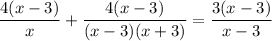
cancel (x - 3)
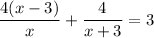
subtract
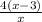 from both sides
from both sides

cross multiply
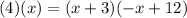
use FOIL
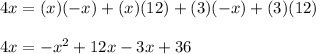
subtract 4x from both sides
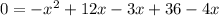
combine like terms

change the signs
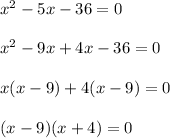
The product is 0 if one of the factors is 0. Therefore:

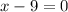 add 9 to both sides
add 9 to both sides
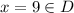
 subtract 4 from both sides
subtract 4 from both sides