Answer:
x = 74.3
Explanation:
Using the trigonometric ratio formula, the missing side, x, of the right angled triangle, can be found as follows:
Ѳ = 22°,
adjacent side length = x
Opposite side length = 30
Thus, we would apply the formula:
tan Ѳ = opposite length/adjacent length
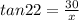
Multiply both sides by x
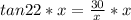
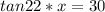

Divide both sides by 0.4040 to make x the subject of the formula
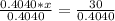

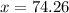
x ≈ 74.3 (to the nearest tenth)