Answer:
0.03333 or 1/30
Explanation:
Let A =" I go sailing" and B = "it does not rain"
P(A) = 0.03
P(B) =0.90
Since I never go sailing when it rains, P(A and B) =0.03.
Therefore, the (conditional) probability that I go sailing, given that it does not rain is:
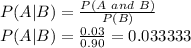
The probability is 0.03333 or 1/30.