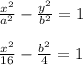Answer:

Explanation:
A hyperbola is the locus of a point such that its distance from a point to two points (known as foci) is a positive constant.
The standard equation of a hyperbola centered at the origin with transverse on the x axis is given as:

The coordinates of the foci is at (±c, 0), where c² = a² + b²
Given that a hyperbola centered at the origin with x-intercepts +/- 4 and foci of +/-2√5. Since the x intercept is ±4, this means that at y = 0, x = 4. Substituting in the standard equation:

The foci c is at +/-2√5, using c² = a² + b²:
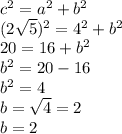
Substituting the value of a and b to get the equation of the hyperbola: