Answer:
a.
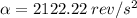
b.

Step-by-step explanation:
The computation is shown below:
data provided in the question
The initial angular velocity
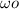 = 0 rev/s,
= 0 rev/s,
f = Final angular velocity =
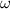 = 382000 rpm i.e =
= 382000 rpm i.e =
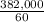 = 6,366.67
= 6,366.67
And time = t = 3.0s
Based on the above information
a. For angular acceleration of drill
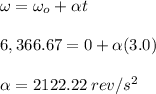
b. For the number of revolutions
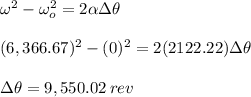
We simply applied the above formulas for determining each parts