Question

The AC voltage source supplies an rms voltage of 146 V at frequency f. The circuit has R = 110 Ω, XL = 210 Ω, and XC = 110 Ω. At the instant the voltage across the generator is at its maximum value, what is the magnitude of the current in the circuit?

Answer 1

1.03A

Step-by-step explanation:

For computing the magnitude of the current in the circuit we need to do the following calculations

LCR circuit impedance

`Z = √(R^2 + (X_L - X_c)^2) \\\\ = √(110^2 + (210 - 110)^2)`

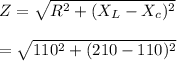
= 148.7Ω

Now the phase angle is

`\phi = tan^(-1) ((X_L - X_C)/(R)) \\\\ = tan^(-1) ((210 - 110)/(110))\\\\ = 42.3^(\circ)`

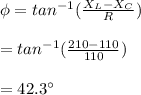
Now the rms current flowing in the circuit is

`I_(rms) = (V_(rms))/(Z) \\\\ = (146)/(148.7)`

= 0.98 A

The current flowing in the circuit is

`I = I_(rms)√(2) \\\\ = (0.98) (1.414)`

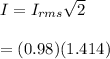
= 1.39 A

And, finally, the current across the generator is

`I'= I cos \phi`

`= (1.39) cos 42.3^(\circ)`

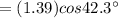
= 1.03A

Hence, the magnitude of the circuit current is 1.03A