Answer:
All possible rational zeros are given by the rational zeros theorem are 1, -1, 19 and -19.
Explanation:
The rational zeros theorem indicates that for a polynomial
f (x) = aₙxⁿ + aₙ₋₁xⁿ⁻ + ⋯ + a₂x² + a₁x + a₀
where aₙ, aₙ₋₁, ⋯ a₀ are integers, the rational roots can be determined from of the factors of aₙ and a₀.
Being p divisor of the independent term a₀ and q divisor of the coefficient principal aₙ, then all rational factors or possible rational roots will take the form of

In the case of p(x)=x³ -7x² +19, aₙ=1 and a₀=19
So:
Dividers of the independent term (19): p=±1,±19
Divisors of the main coefficient (1): q=±1
Being the possible roots of the polynomial:
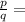 ±1, ±19
±1, ±19
All possible rational zeros are given by the rational zeros theorem are 1, -1, 19 and -19.