Answer:
The width (side perpedicular to the wall of the werehouse): x = 30 m
The lenght (side parallel to the wall of the werehouse): y = 60 m
Explanation:
x - the width of the fenced area
Randy has 120 m of fencing so for the lenght remain (120-2x) m of fencing:
y = 120 - 2x
Area of the fencing: A = x•y
A(x) = x•(120 - 2x)
A(x) = -2x² + 120x ← quadratic function
The maximum value of quadratic function occurs at:
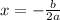
a = -2 b = 120
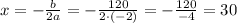
y = 120 - 2•30 = 60