Question

How do I solve this?

Answer 1

See below.

Explanation:

`(5x^2y^3)^0/(-2x^(-3)y^5)^(-2)`

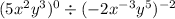
First, note that everything to the zeroth power is 1. Thus:

`=1/(-2x^(-3)y^5)^(-2)=(1)/((-2x^(-3)y^5)^(-2))`

Distribute using Power of a Power property:

`=(1)/((-2)^(-2)(x^(-3))^(-2)(y^5)^(-2)))`

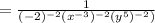
Make the exponents positive by putting them to the numerator:

`=((-2)^2(x^(-3))^2(y^5)^2)/(1)`

`=(4x^(-6)y^(10))/(1)`

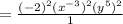
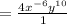
Make the exponent positive by this time putting it to the denominator:

`=(4y^(10))/(x^6)`