Question

To construct a solenoid, you wrap insulated wire uniformly around a plastic tube 7.1 cm in diameter and 57 cm in length. You would like a 3.0 A current to produce a 2.6 kG magnetic field inside your solenoid. What is the total length of wire you will need to meet these specifications?

Answer 1

We need about 8769 meters of wire to produce a 2.6 kilogauss magnetic field.

Step-by-step explanation:

Recall the formula for the magnetic field produced by a solenoid of length L. N turns, and running a current I:

`B=\mu_0\,(N)/(L) \,I`

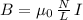
So, in our case, where B = 2.6 KG = 0.26 Tesla; I is 3 amperes, and L = 0.57 m, we can find what is the number of turns needed;

`B=\mu_0\,(N)/(L) \,I\\0.26=4\,\pi\,10^(-7)(N)/(0.57) \,3\\N=(0.26*0.57\,10^7)/(12\,\pi) \\N=39311.27`

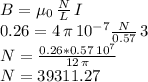
Therefore we need about 39312 turns of wire. Considering that each turn must have a length of
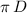`\pi\,D`, where D is the diameter of the plastic cylindrical tube, then the total length of the wire must be:

`Length=39312\,(\pi\,D)=39312\,(\pi\,0.071)\approx 8768.66\,\,m`

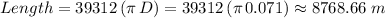
We can round it to about 8769 meters.