Question

In a clinical study of an allergy drug, 108 of the 200 subjects reported experiencing significant relief from their symptoms. Test the claim that more than half of all those using the drug experience relief. Using a 0.01 significance level, what is your decision

Answer 1

The decision is to not reject the null hypothesis.

At a significance level of 0.01, there is not enough evidence to support the claim that the proportion of all those using the drug that experience relief is significantly higher than 50% (P-value = 0.1443).

Explanation:

This is a hypothesis test for a proportion.

The claim is that the proportion of all those using the drug that experience relief is significantly higher than 50%.

Then, the null and alternative hypothesis are:

`H_0: \pi=0.5\\\\H_a:\pi>0.5`

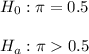
The significance level is 0.01.

The sample has a size n=200.

The sample proportion is p=0.54.

`p=X/n=108/200=0.54`

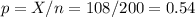
The standard error of the proportion is:

`\sigma_p=\sqrt{(\pi(1-\pi))/(n)}=\sqrt{(0.5*0.5)/(200)}\\\\\\ \sigma_p=√(0.00125)=0.035`

Then, we can calculate the z-statistic as:

`z=(p-\pi-0.5/n)/(\sigma_p)=(0.54-0.5-0.5/200)/(0.035)=(0.038)/(0.035)=1.061`

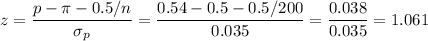
This test is a right-tailed test, so the P-value for this test is calculated as:

`\text{P-value}=P(z>1.061)=0.1443`

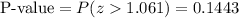
As the P-value (0.1443) is greater than the significance level (0.01), the effect is not significant.

The null hypothesis failed to be rejected.

At a significance level of 0.01, there is not enough evidence to support the claim that the proportion of all those using the drug that experience relief is significantly higher than 50%.