Question

Which function has the greatest rate of exponential growth? Multiple choice options below.

Answer 1

Option (3).(Bottom left)

Explanation:

Formula for the exponential growth is,

h(x) =
`a(1+(r)/(100) )^(t)`

where r = rate of exponential growth

a = initial amount

t = duration or time

Option (1) (Top left)

h(t) =
`(1+0.18)^{(t)/(6)}`

=
`(1+(18)/(100))^t`

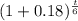
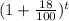
Rate of exponential growth will be,

r = 18%

Option (2) (Top right)

k(t) =
`((3)/(8))^t`

=
`(1-(5)/(8))^t`

=
`(1-0.625)^t`

=
`(1-(62.5)/(100))^t`

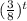
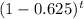
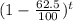
r = -62.5%

This function shows the exponential decay rate of 62.5%.

Option (3) (Bottom left)

f(t) =
`1.36^t`

=
`(1+0.36)^t`

=
`(1+(36)/(100))^t`

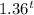
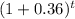
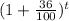
r = 36%

Option (4) (Bottom right)

g(t) =
`0.86^t`

=
`(1-0.14)^t`

=
`(1-(14)/(100))^t`

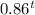
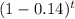
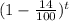
r = -14%

This function shows the decay rate of 14%.

Therefore, Option (3). (Bottom left) shows the greatest exponential growth.