Question

Study the diagram of circle V, where two chords, JK¯¯¯¯¯¯¯¯ and LM¯¯¯¯¯¯¯¯¯, are equidistant from the center.

If JK=3x+19 and LM=6x+7, what is the length of segment JK?


15.5

4

24

31

3

Answer 1

Option (4). 31

Explanation:

Although the figure attached is not clear, but the question itself is self explanatory.

Theorem of a circle says,

"Two chords equidistant from the center of a circle, measure the same in length."

Therefore, JK ≅ LM

m(JK) = m(LM)

3x + 19 = 6x + 7

3x - 6x = 7 - 19

-3x = -12

x =
`(12)/(3)`

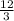
x = 4

m(JK) = 3x + 19

= 3(4) + 19

= 12 + 19

= 31

Therefore, length of JK is 31 units.

Option (4) will be the answer.