Question

You bought one of Bergen Manufacturing Co.’s 7.8 percent coupon bonds one year ago for $1,061. These bonds make annual payments and mature twelve years from now. Suppose you decide to sell your bonds today when the required return on the bonds is 4.5 percent. If the inflation rate was 4.4 percent over the past year, what would be your total real return on the investment?

Answer 1

The answer is "24.48%"

Step-by-step explanation:

Given:

current Bond Value is PV(0.045,12,78,1000) = 1300.91

rate = 0.045

time =
`(n)/(12)`

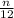
coupon number = pmt = 78

price = fv=1000

Total profit = 1300.91 -1061

= 239.91.

Throughout,

The year a bondholder got we should include a coupon rate =78.

So, profit:

`= 239.91 + 78 \\\\ = 317.91`

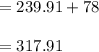
return:

`=(317.91)/(1061)\\\\ = 29.96 %`

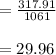
The actual return on investment accrued to both the formula of brandt is

`=((nr-ir))/((1+ir))* 100 _(where)\\\\ nr = \texttt{nominal rate}\\\\ ir = \texttt{inflation rate} \\\\ nr = 29.96\% \\\\ ir = 4.4\%\\`

by replacing the formula would result in a real return rate of 24.48 %