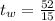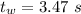Question

A hot air balloon competition requires a balloonist to drop a ribbon onto a target on the ground. Initially the hot air balloon is 50 meters above the ground and 100 meters from the target. The wind is blowing the balloon at v = 15 meters/sec on a course to travel directly over the target. The ribbon is heavy enough that any effects of the air slowing the vertical velocity of the ribbon are negligible. How long should the balloonist wait to drop the ribbon so that it will hit the target?

time =

Answer 1

The waiting time is
`t_w = 3 .47 \ s`

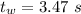
Step-by-step explanation:

From the question we are told that

The height of the hot air balloon above the ground is
`d = 50 \ m`

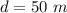
The distance of the balloon from the target is
`l = 100 \ m`

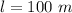
The velocity of the balloon is
`v = 15 \ m/s`

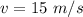
Generally the time it will take to reach the ground is

`t = \sqrt{2 * (d)/(g) }`

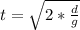
substituting values

`t = \sqrt{2 * (50)/(9.8) }`

`t = 3.2 \ s`

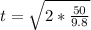
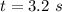
The distance that is covered at time with the given velocity is mathematically evaluated as

`z = v * t`

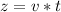
substituting values

`z = 15 * 3.2`

`z = 48 \ m`

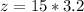
This implies that for the balloon moving at a velocity (v) to hit the target it must be dropped at this distance (z)

Now the distance the balloonist has to wait before dropping in order to hit the target is

`A = d - z`

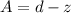
substituting values

`A = 100 - 48`

`A = 52 \ m`

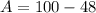
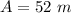
This implies that the time the balloonist has to wait is

`t_w = (A)/(v)`

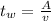
substituting values

`t_w = (52)/(15)`

`t_w = 3 .47 \ s`