Question

X^2 - 12x + 19 in the form of (x + a)^2 + b ???

Answer 1

( x-6)^2 -17

Explanation:

X^2 - 12x + 19

we need it in given below form

(x + a)^2 + b

we know that

`(x + a)^2 = x^2 + 2ax + a^2\\`

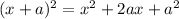
lets convert that in same form

X^2 - 12x + 19 = x^2 + 2(-6)x + 19

comparing x^2 + 2(-6)x to x^2 + 2ax

we have a -6

now

(x-6)^2 = x^2 + 2(-6)x + 36

36 is missing in the x^2 + 2(-6)x + 19 hence to get that

we add and subtract 36 in the above equation

so we have

x^2 + 2(-6)x + 19 + 36 -36

rearranging it \

(x^2 + 2(-6)x + 36) -36 + 19 (x^2 + 2(-6)x + 36 =( x-6)^2)

=>( x-6)^2 -17

comparing the above equation to

(x + a)^2 + b

we have a = -6

b = -17

( x-6)^2 -17