Answer:
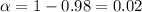
And
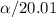 , the degrees of freedom are given by:
, the degrees of freedom are given by:
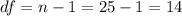
Then the critical value using the t distribution with 24 degrees of freedom is:
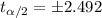
And the best solution would be:
0 -2.492
Explanation:
For this problem we know that the sample size is n = 25. The confidence level is 98% or 0.98 then the significance would be:
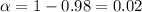
And
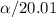 , the degrees of freedom are given by:
, the degrees of freedom are given by:
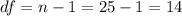
Then the critical value using the t distribution with 24 degrees of freedom is:
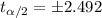
And the best solution would be:
0 -2.492