Question

Find the product of all positive integer values of $c$ such that $3x^2+7x+c=0$ has two real roots. I will award a lot of points

Answer 1

$3x^2+7x+c=0$

comparing above equation with ax²+bx+c

a=3

b=7

c=1

using quadratic equation formula

`x = \frac{ - b + - \sqrt{b {}^(2) - 4ac} }{ 2a}`

x=(-7+-√(7²-4×3×1))/(2×3)

x=(-7+-√13)/6

taking positive

x=(-7+√13)/6=

taking negative

x=(-7-√13)/6=

Answer 2

Answer: 24

Explanation:

Let's find one solution:

3x² + 7x + c = 0

a=3 b=7 c=c

First, let's find c so that it has REAL ROOTS.

⇒ Discriminant (b² - 4ac) ≥ 0

7² - 4(3)c ≥ 0

49 - 12c ≥ 0

-12c ≥ -49

`c\leq(-49)/(-12)\quad \rightarrow c\leq (49)/(12)`

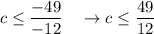
Since c must be a positive integer, 1 ≤ c ≤ 4

Example: c = 4

3x² + 7x + 4 = 0

(3x + 4)(x + 1) = 0

x = -4/3, x = -1 Real Roots!

You need to use Quadratic Formula to solve for c = {1, 2, 3}

Valid solutions for c are: {1, 2, 3, 4)

Their product is: 1 x 2 x 3 x 4 = 24