Question

A line segment has endpoints at (8, 3) and (2,5). What would be the equation of this line's perpendicular bisector?

Answer 1

y = −1/3x+17/3

Explanation:

The line segment has slope -1/3. This means that any line perpendicular to it will have a slope of 3 (negative reciprocal)

Any line that bisects the line segment will pass through its midpoint. The midpoint is (5,4)

Midpoint formula:
`( (x_1+x_2)/(2), (y_1+y_2)/(2) )`

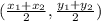
So perpendicular bisector of this line is simply a line with slope −1/3 that passes through point (5, 4)

y - 4 = -1/3 (x-5)=

y = −1/3x+17/3