Question

A simple random sample of 44 adults is obtained from a normally distributed​ population, and each​ person's red blood cell count​ (in cells per​ microliter) is measured. The sample mean is 5.31 and the sample standard deviation is 0.51 . Use a 0.05 significance level and the given calculator display to test the claim that the sample is from a population with a mean less than 5.4 comma which is a value often used for the upper limit of the range of normal values. What do the results suggest about the sample​ group?

Answer 1

There is not enough evidence to support the claim that the population mean is significantly less than 5.4.

This result suggest we may be making a Type II error, where a true alternative hypothesis does not have enough evidence to be supported.

If the same outcome would have been obtained with a bigger sample size, the power of the test is bigger and there is a higher probability of rejecting the null hypothesis.

Explanation:

This is a hypothesis test for the population mean.

The claim is that the population mean is significantly less than 5.4.

Then, the null and alternative hypothesis are:

`H_0: \mu=5.4\\\\H_a:\mu< 5.4`

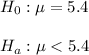
The significance level is 0.05.

The sample has a size n=44.

The sample mean is M=5.31.

As the standard deviation of the population is not known, we estimate it with the sample standard deviation, that has a value of s=0.51.

The estimated standard error of the mean is computed using the formula:

`s_M=(s)/(√(n))=(0.51)/(√(44))=0.077`

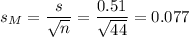
Then, we can calculate the t-statistic as:

`t=(M-\mu)/(s/√(n))=(5.31-5.4)/(0.077)=(-0.09)/(0.077)=-1.171`

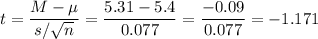
The degrees of freedom for this sample size are:

`df=n-1=44-1=43`

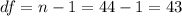
This test is a left-tailed test, with 43 degrees of freedom and t=-1.171, so the P-value for this test is calculated as (using a t-table):

`\text{P-value}=P(t<-1.171)=0.124`

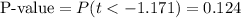
As the P-value (0.124) is bigger than the significance level (0.05), the effect is not significant.

The null hypothesis failed to be rejected.

There is not enough evidence to support the claim that the population mean is significantly less than 5.4.

This result suggest we may be making a Type II error, where a true alternative hypothesis does not have enough evidence to be supported.

If the same outcome would have been obtained with a bigger sample size, the power of the test is bigger and there is a higher probability of rejecting the null hypothesis.