Question

QT and PM

trianglePQR. If QR = 8 cm, PR 7 cm and
QT = 4 cm, what is PM?
P=7 cm
M=8 cm​

Answer 1

The length of the altitude PM is 3.5 cm.

Explanation:

We are given that QT and PM are the altitudes of the triangle PQR. Also, QR = 8 cm, PR = 7 cm and QT = 4 cm.

We have to find the length of the altitude PM.

As we know that the area of the triangle is given by;

Area of triangle =
`(1)/(2) * \text{Base} * \text{Height(Altitude)}`

Here, in
`\triangle`PQR; Base = PR = 7 cm

Height(Altitude) = QT = 4 cm

So, the area of the triangle PQR =
`(1)/(2) * 7 * 4`

= 14 sq cm.

Similarly, the area of the triangle can also be;

Area =
`(1)/(2) * \text{QR} * \text{PM}`

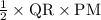
Here, QR = Base of triangle PQR = 8 cm

PM = the required altitude

So, Area of triangle =
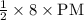
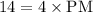
`(1)/(2) * 8* \text{PM}`

`14 =4 * \text{PM}`

PM =
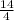`(14)/(4)` = 3.5 cm

Hence, the length of the altitude PM is 3.5 cm.