Question

A cell membrane consists of an inner and outer wall separated by a distance of approximately 10 nm. Assume that the walls act like a parallel plate capacitor, each with a charge density of 10^(−5) C/m^2, and the outer wall is positively charged. Although unrealistic, assume that the space between cell walls is filled with air. What is the magnitude of the electric field between the membranes?ε0=8.85x10^(-12) C^2/(Nm^2)

Answer 1

E = 1.29*10^6N/C

Step-by-step explanation:

You take the cell membrane as a parallel plate capacitor. In order to calculate the magnitude of the electric field in between the membranes you use the following formula:

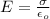`E=(\sigma)/(\epsilon_o)` (1)

σ: surface charge density = 10^-5 C/m^2

εo: dielectric permittivity of vacuum = 8.85*10^-12C^2/Nm^2

You replace the values of the parameters in the equation (1):

`E=(10^(-5)C/Nm^2)/(8.85*10^(-12)C^2/Nm^2)=1.29*10^6(N)/(C)`

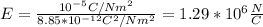
The magnitude of the electric field between the membrane cell is 1.29*10^6N/C