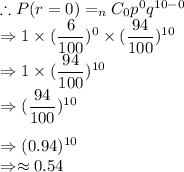Question

The probability that a house in an urban area will be burglarized is 6​%. If 10 houses are randomly​ selected, what is the probability that none of the houses will be​ burglarized?

Answer 1

`((94)/(100))^(10) \ or\ \approx 0.54`

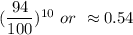
Explanation:

Given :

Probability that a house in an urban area will be burglarized,

`p =6\%=(6)/(100)`

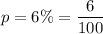
To find:

Probability that none of the houses randomly selected from 10 houses will be burglarized = ?

`P(r=0) =?`

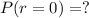
Solution:

This question is related to binomial distribution where:

`p =(6)/(100)`

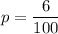
`\Rightarrow` Probability that a house in an urban area will not be burglarized,

`q =1-6\%=94\%=(94)/(100)`

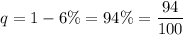
Formula is:

`P(r=x)=_nC_xp^xq^(n-x)`

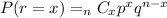
Where n is the total number of elements in sample space and

x is the number selected from the sample space.

Here, x = 10 and

x = 0

`\therefore P(r=0)=_nC_0p^0q^(10-0)\\\Rightarrow 1 * ((6)/(100))^0* ((94)/(100))^(10)\\\Rightarrow 1* ((94)/(100))^(10)\\\Rightarrow ((94)/(100))^(10)\\\\\Rightarrow (0.94)^(10)\\\Rightarrow \approx 0.54`