Question

In the 25 ftft Space Simulator facility at NASA's Jet Propulsion Laboratory, a bank of overhead arc lamps can produce light of intensity 2500 W/m^2 at the floor of the facility. (This simulates the intensity of sunlight near the planet Venus.)

Required:
Find the average radiation pressure (in pascals and in atmospheres) on

a. A totally absorbing section of the floor.
b. A totally reflecting section of the floor.
c. Find the average momentum density (momentum per unit volume) in the light at the floor.

Answer 1

a) 8.33 x
`10^(-6)` Pa or 8.22 x
`10^(-11)` atm

b) 1.66 x
`10^(-5)` Pa or 1.63 x
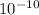`10^(-10)` atm

c) 2.77 x
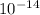`10^(-14)` kg/m^2-s

Step-by-step explanation:

Intensity of light = 2500 W/m^2

area = 25 ft^2

a) average radiation pressure on a totally absorbing section of the floor
`Pav = (I)/(c)`

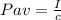
where I is the intensity of the light

c is the speed of light =
`3*10^(8) m/s`

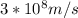
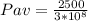`Pav = (2500)/(3*10^(8) )` = 8.33 x
`10^(-6)` Pa

1 pa =
`9.87*10^(-6)`

8.33 x
`10^(-6)` Pa = 8.22 x
`10^(-11)` atm

b) average radiation for a totally radiating section of the floor

`Pav = (2I)/(c)`

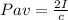
this means that the pressure for a totally radiating section is twice the average pressure of the totally absorbing section

therefore,

Pav = 2 x 8.33 x
`10^(-6)` = 1.66 x
`10^(-5)` Pa

or

Pav in atm = 2 x 8.22 x
`10^(-11)` = 1.63 x
`10^(-10)` atm

c) average momentum per unit volume is

`m = (I)/(c^(2) )`

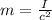
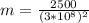`m = (2500)/((3*10^(8)) ^(2) )` = 2.77 x
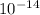`10^(-14)` kg/m^2-s