Answer:
4x + 6
Explanation:
Given
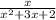 +
+
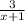
Before we can add the fractions we require them to have a common denominator.
Factor the denominator of the first fraction
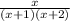 +
+
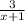
Multiply the numerator / denominator of the second fraction by (x + 2)
=
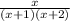 +
+
 ← fractions now have a common denominator
← fractions now have a common denominator
Add the numerators leaving the denominators
=
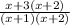
=

=
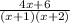 ← simplified sum with numerator 4x + 6
← simplified sum with numerator 4x + 6