Question

Angie and Brian are running a bakery. They are going to make three kinds of banana bread: regular, one with extra sugar, and one with extra bananas. Each loaf of regular takes 3 bananas, 3 cups of sugar, and 2 cups of flour. Each loaf of extra sugar takes 2 bananas, 4 cups of sugar, and 3 cups of flour. Each loaf of extra bananas takes 5 bananas, 1 cup of sugar and 4 cups of flour. They have 1200 bananas, 1050 cups of sugar, and 1075 cups of flour available. How many loaves of each type should they make to use up all the bananas, sugar and flour available

Answer 1

150 regular loaves

125 loaves with extra sugar

100 loaves with extra bananas.

Explanation:

Let R represent loaves of regular bread, S represent loaves with extra sugar, and B represent loaves with extra bananas. If they have 1200 bananas, 1050 cups of sugar, and 1075 cups of flour available, the number of each type of bread that Angie and Brian can make are given by the following system of equations:

`3R+2S+5B=1,200\\3R+4S+1B=1,050\\2R+3S+4B=1,075`

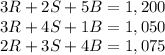
Solving the linear system:

`3R+4S+1B-(3R+2S+5B)=1,050-1,200\\2S-4B=-150\\S=-75+2B\\\\3R+2*(-75+2B)+5B=1,200\\2R+3*(-75+2B)+4B=1,075\\3R+9B=1,350\\2R+10B=1,300\\\\3R+9B-1.5*(2R+10B)=1,350-(1.5*1,300)\\9B-15B=-600\\B=100\\2R+10*100=1,300\\R=150\\3*150+2S+5*100=1,200\\S=125`

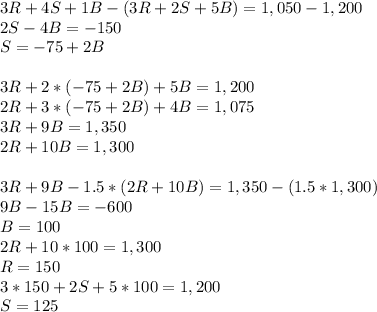
They should make 150 regular loaves, 125 loaves with extra sugar, and 100 loaves with extra bananas.