Answer:
16.10 kJ
Step-by-step explanation:
The thermal energy created in the slope can be found by definition of work (W):
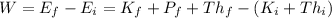
Where:
 and
and
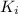 : is the final and initial kinetic energy
: is the final and initial kinetic energy
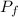 : is the final potential energy
: is the final potential energy
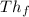 and
and
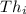 : is the final and initial thermal energy
: is the final and initial thermal energy
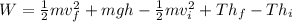
We have that W is:
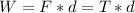
Where:
F: is the force equal to the tension (T)
d: is the displacement = 120 m
And since the speed is constant,
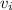 =
=
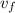 we have:
we have:
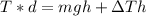
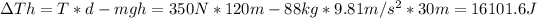
Therefore, the thermal energy created in the slope and the tube during the ascent is 16.10 kJ.
I hope it helps you!