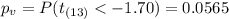Question

A safety officer wants to prove that μ = the average speed of cars driven by a school is less than 25 mph. Suppose that a random sample of 14 cars shows an average speed of 24.0 mph, with a sample standard deviation of 2.2 mph. Assume that the speeds of cars are normally distributed. What is the p-value?

Answer 1

`t=(24-25)/((2.2)/(√(14)))=-1.70`

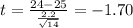
The degrees of freedom are given by:

`df=n-1=14-1=13`

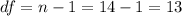
The p value for this case would be given by:

`p_v =P(t_((13))<-1.70)=0.0565`

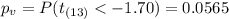
Explanation:

Information given

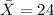`\bar X=24` represent the mean height for the sample

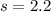`s=2.2` represent the sample standard deviation

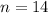`n=14` sample size

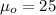`\mu_o =25` represent the value that we want to test

t would represent the statistic

`p_v` represent the p value for the test

Hypothesis to verify

We want to cehck if the true mean is lees than 25 mph, the system of hypothesis would be:

Null hypothesis:
`\mu \geq 25`

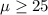
Alternative hypothesis:
`\mu < 25`

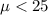
The statistic would be given by:

`t=(\bar X-\mu_o)/((s)/(√(n)))` (1)

Replacing the info given we got:

`t=(24-25)/((2.2)/(√(14)))=-1.70`

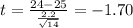
The degrees of freedom are given by:

`df=n-1=14-1=13`

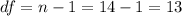
The p value for this case would be given by:

`p_v =P(t_((13))<-1.70)=0.0565`