Question

To test whether or not there is a difference between treatments A, B, and C, a sample of 12 observations has been randomly assigned to the three treatments. You are given the results below. Treatment Observation A 20 30 25 33 B 22 26 20 28 C 40 30 28 22 The test statistic to test the null hypothesis equals _____.

Answer 1

The test statistic to test the null hypothesis equals 1.059

Explanation:

From the given information; we have:

Treatment Observations

A 20 30 25 33

B 22 26 20 28

C 40 30 28 22

The objective is to find the test statistic to test the null hypothesis; in order to do that;we must first run through a series of some activities.

Let first compute the sum of the square;

Total sum of squares (TSS) = Treatment sum of squares
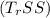`(T_r SS)` + Error sum of squares (ESS)

where:

(TSS) =
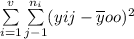`\sum \limits ^v_(i=1) \sum \limits ^(n_i)_(j-1)(yij- \overline {y}oo)^2` with (n-1) df

`(T_r SS)`
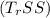
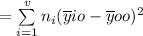`= \sum \limits ^v_(i=1) n_i( \overline yio- \overline {y}oo)^2` with (v-1) df

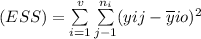`(ESS) = \sum \limits ^v_(i=1) \sum \limits ^(n_i)_(j-1)(yij- \overline {y}io)^2` with (n-v) df

where;

v= 3

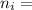`n_i=`4

i = 1,2,3

n =12

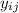`y_(ij)` is the
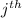`j^{th` observation for the
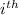`i^{th` treatment

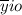`\overline{y}io` is the mean of the
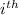`i^{th` treatment i = 1,2,3 ; j = 1,2,3,4

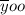`\overline y oo` is the overall mean

From the given data

`\overline y oo = (1)/(12) \sum \limits ^3_(i=1) \sum \limits ^(4)_(j=1)(yij)^2= 27`

`TSS = (1)/(12) \sum \limits ^3_(i=1) \sum \limits ^(4)_(j=1)(yij- 27)^2 = 378`

`T_r SS= \sum \limits^3_(i=1)4 (\overline y io - \overline yoo)^2`

`=4(27-27)^2+4(24-27)^2+4(30-27)^2 = 72`

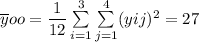
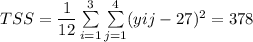
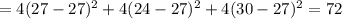
Total sum of squares (TSS) = Treatment sum of squares
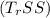`(T_r SS)` + Error sum of squares (ESS)

(TSS) = 378 - 72

(TSS) = 306

Now; to the mean square between treatments (MSTR); we use the formula:

MSTR = TrSS/df(TrSS)

MSTR = 72/(3 - 1)

MSTR = 72/2

MSTR = 36

The mean square within treatments (MSE) is:

MSE = ESS/df(ESS)

MSE = 306/(12-3)

MSE = 306/(9)

MSE = 34

The test statistic to test the null hypothesis is :

`T = ( (TrSS)/(\sigma^2)/(v-1) )/( (ESS)/(\sigma^2)/(n-v) ) = (MSTR)/(MSE) \ \ \ \approx \ \ T(\overline {v-1}, \overline {n-v})`

`T = (36)/(34)`

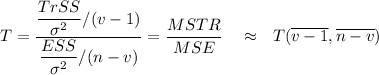
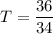
T = 1.059