Question

The following data show the brand, price , and the overall score for stereo headphones that were tested by Consumer Reports. The overall score is based on sound quality and effectiveness of ambient noise reduction. Scores range from (lowest) to (highest). The estimated regression equation for these data is = 23.194 + 0.318x, where x = price ($) and y = overall score.

Brand Price Score
Bose 180 76
Scullcandy 150 71
Koss 95 62
Phillips/O'Neill 70 57
Denon 70 30
JVC 35 34

Required:
a. Compute SST, SSR, and SSE (to 3 decimals).
b. Compute the coefficient of determination r2.
c. What is the value of the sample correlation coefficient?

Answer 1

a. SST = 1816

SSR = 1511.804

SSE = 465.804

b. Coefficient of determination, R² = 0.832491079

c. The correlation coefficient r = 0.8636

Explanation:

y = 23.194 + 0.318·x

Where:

x = Price

y = Overall score

The observed data are given as follows;

Brand Price Score

Bose 180 76

Scullcandy 150 71

Koss 95 62

Phillips/O'Neill 70 57

Denon 70 30

JVC 35 34

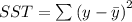`SST = \sum \left (y - \bar{y} \right )^(2)`= 1816

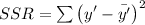`SSR = \sum \left ({y}'-\bar{y{}'} \right )^(2)` = 1511.804

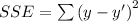`SSE = \sum \left (y - {y}' \right )^(2)` = 465.804

Coefficient of determination

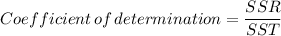`Coefficient \, of \, determination = (SSR)/(SST)`= 0.832

Coefficient of correlation =

`r = \frac{n\left (\sum xy \right )-\left (\sum x \right )\left (\sum y \right )}{\sqrt{\left [n\sum x^(2)-\left (\sum x \right )^(2) \right ]\left [n\sum y^(2)-\left (\sum y \right )^(2) \right ]}}`

Ʃxy = 37500

Ʃx =600

Ʃy = 330

Ʃx² = 74950

Ʃy² = 19966

`r = \frac{6 \left (37500 \right )-\left (600 \right )\left (330 \right )}{\sqrt{\left [6* 74950-\left (600 \right )^(2) \right ]\left [6 * 19966-\left (330 \right )^(2) \right ]}} = 0.8636`