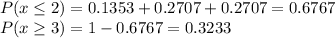Question

7. Defects in poured metal caused by contamination follows a Poisson distribution with average number of occurrences being 2 per cubic millimeter. What is the probability that there will be at least three defects in a randomly selected cubic millimeter of this metal

Answer 1

`P(x\geq 3)=0.3233`

Explanation:

If the number of defects in poured metal follows a Poisson distribution, the probability that x defects occurs is:

`P(x)=(e^(-m)*(m)^(x))/(x!)`

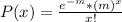
Where x is bigger or equal to zero and m is the average. So replacing m by 2, we get that the probability is equal to:

`P(x)=(e^(-2)*(2)^(x))/(x!)`

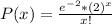
Finally, the probability that there will be at least three defects in a randomly selected cubic millimeter of this metal is equal to:

`P(x\geq 3)=1-p(x\leq 2)\\`

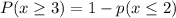
Where
`P(x\leq 2)=P(0)+P(1)+P(2)`

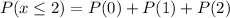
So, P(0), P(1) and P(2) are equal to:

`P(0)=(e^(-2)*(2)^(0))/(0!)=0.1353\\P(1)=(e^(-2)*(2)^(1))/(1!)=0.2707\\P(2)=(e^(-2)*(2)^(2))/(2!)=0.2707`

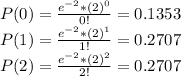
Finally,
`P(x\leq2)` and
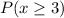`P(x\geq3)` are equal to:

`P(x\leq 2)=0.1353+0.2707+0.2707=0.6767\\P(x\geq 3)=1-0.6767=0.3233`