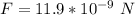Answer:
The electric force is
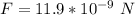
Step-by-step explanation:
From the question we are told that
The Bohr radius at ground state is
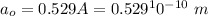
The values of the distance between the proton and an electron
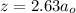
The electric force is mathematically represented as

Where n and p are charges on a single electron and on a single proton which is mathematically represented as
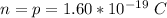
and k is the coulomb's constant with a value
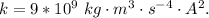
substituting values
![F = (9*10^(9) * [(1.60*10^(-19) ]^2))/((2.63 * 0.529 * 10^(-10))^2)](https://img.qammunity.org/2021/formulas/physics/college/37kep8n5vlxui50f0cklw0vpuc5f0svkft.png)