Question

A Young's interference experiment is performed with blue-green laser light. The separation between the slits is 0.500 mm, and the screen is located 3.24 m from the slits. The first bright fringe is located 3.30 mm from the center of the interference pattern. What is the wavelength of the laser light?

Answer 1

λ = 509 nm

Step-by-step explanation:

In order to calculate the wavelength of the light you use the following formula:

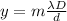`y=m(\lambda D)/(d)` (1)

where

y: distance of the mth fringe to the central peak = 3.30 mm = 3.30*10^-3 m

m: order of the bright fringe = 1

D: distance from the slits to the screen = 3.24 m

d: distance between slits = 0.500 mm = 0.500*10^-3 m

You first solve the equation (1) for λ, and then you replace the values of the other parameters:

`\lambda=(dy)/(mD)\\\\\lambda=((0.500*10^(-3)m)(3.30*10^(-3)m))/((1)(3.24m))=5.09*10^7m\\\\\lambda=509*10^(-9)m=509nm`

The wavelength of the light is 509 nm