Answer:
C.Quadrilateral ABCD is not a rhombus because there are no pairs of parallel sides.
Complete question:
A. Quadrilateral ABCD is not a rhombus because opposite sides are parallel but the four sides do not all have the same length.
B. Quadrilateral ABCD is a rhombus because opposite sides are parallel and all four sides have the same length.
C. Quadrilateral ABCD is not a rhombus because there are no pairs of parallel sides.
D. Quadrilateral ABCD is not a rhombus because there is only one pair of opposite sides that are parallel.
Explanation:
Rhombus states that a parallelogram with four equal sides and sometimes one with no right angle.
Given: The coordinate of the vertices of quadrilateral ABCD are A(−6, 3) , B(−1, 5) , C(3, 1) , and D(−2, −2) .
The condition for the segment
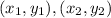 to be parallel to
to be parallel to
 is matching slopes;
is matching slopes;
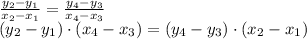 ---->1
---->1
So, we have to check that AB || CD and AD || BC
First check AB || CD
A(−6, 3) , B(−1, 5) , C(3, 1) , and D(−2, −2)
substitute in [1],
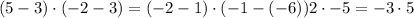
-10 ≠ -15
Similarly,
check AD || BC
A(−6, 3) , D(−2, −2) , B(−1, 5) and C(3, 1)
Substitute in [1], we have
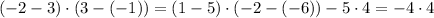
-20 ≠ -16.
Both pairs of sides are not parallel,
therefore, Quadrilateral ABCD is not a rhombus because there are no pairs of parallel sides.