Question

Find the area of the trapezoid to the nearest tenth.

Answer 1

2.2 metres squared

Explanation:

We need to find the area of this trapezoid.

The area of a trapezoid is denoted by:

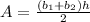`A=((b_1+b_2)h)/(2)`, where
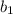`b_1` and
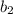`b_2` are the parallel bases and h is the height

Here, we already know the lengths of the two bases; they are 0.9 metres and 2.3 metres. However, we need to find the length of the height.

Notice that one of the angles is marked 45 degrees. Let's draw a perpendicular line from top endpoint of the segment labelled 0.9 to the side labelled 2.3. We now have a 45-45-90 triangle with hypotenuse 2.0 metres. As one of such a triangle's properties, we can divide 2.0 by √2 to get the length of both legs:

2.0 ÷ √2 = √2 ≈ 1.414 ≈ 1.4

Thus, the height is h = 1.4 metres. Now plug all these values we know into the equation to find the area:

`A=((b_1+b_2)h)/(2)`

`A=((0.9+2.3)*1.4)/(2)=2.2`

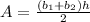
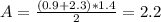
The answer is thus 2.2 metres squared.

~ an aesthetics lover