Answer:
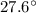
Explanation:
Given:
A
 with
with

ST = 44 feet
TR = 84 feet
To find:
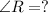
Solution:
Please have a look at the attached figure for clear view of the given dimensions of the right angled triangle.
Here, we can use trigonometric formula to find out the
 .
.
We know that formula for tangent of angle
 is given as:
is given as:
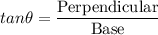
Here, Perpendicular for
 is side ST and
is side ST and
Base is side TR.
Putting the values in above tangent formula:
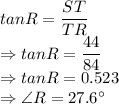
Hence,
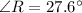 is the answer.
is the answer.