Question

Anyone know how to do #14 and #15?????

Answer 1

He Melanie , use SOH CAH TOA to remember the trig functions

Explanation:

for 14 they hyp = 22 and we know one angle of 45°, we also know that the triangle has a right angle or 90° and by inspection we can tell that the other angle is also going to be 45° so we know X and Y will be the same length, is why this is a good observation.

Use one of the trig functions with H in it b/c you know Hyp is 22, so,

SOH = Sin(Θ)= Opp / Hyp

Sin(45) = Opp / 22

Sin(45) * 22 = Opp

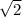`√(2)` / 2 * 22 = opp

11*
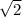`√(2)` = opp is the exact answer, if you would like some decimal places, it's 7.7781 units, both X and Y

for 15 we can use SOH again for the bigger triangle. we know the Hyp is 24 and the angle is 30°

Sin(30) = Opp / 24

Sin(30) *24 = Opp

12 = Opp ( Because I know Sin(30) = 1/2 )

Opp is the upright between the two triangles

Use CAH Cos(Θ) = Adj / Hyp

Cos(30) = Adj / 24

Cos(30) * 24 = Adj

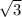`√(3)` /2 *24 =Adj

12
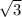`√(3)` = Adj

Z = 12
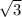`√(3)` or if you want some decimals 20.7846

Becasue the smaller triangle is also a 45° right triangle we know that the upright and side Y are the same lengths

then

Y = 12

You could use SOH or CAH to find the Hyp but, lets just resort to Pythagoras, and use his formula to find Hyp

Hyp =
`\sqrt{12^(2) +12^(2) }`

Hyp =
`√(288)`

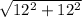
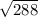
hyp = 16.97

X = 16.97

:)