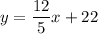Question

Write the slope-intercept form of the equation of the line that is perpendicular to and passes through Point X. Show all work for full credit.

Answer 1

`y =(12)/(5)x + 22`

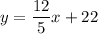
Explanation:

Slope-intercept form of line:

First find the slope of the line AB. ie, m

Slope of the perpendicular line = -1/m

(2 , 3) ⇒ x₁ = 2 & y₁ = 3

(-10, 8) ⇒ x₂ = -10 & y₂ = 8

`\boxed{Slope=(y_2-y_1)/(x_2-x_1)}`

`= (8-3)/(-10-2)\\\\=(5)/(-12)\\\\=(-5)/(12)`

`\sf slope \ of \ the \ perpendicular \ line \ m_1 = (-1)/(m)= -1 \ / (-5)/(12)`

`\sf = -1 * (12)/(-5)=(12)/(5)`

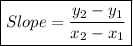
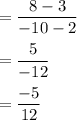
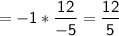
Equation of the required line: y = mx + b

`y =(12)/(5)x+b`

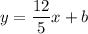
The line passes through (-5 , 10). Substitute in the above equaiton,

`10 =(12)/(5)*(-5) + b\\\\ 10 = (-12) + b\\\\`

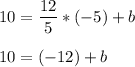
10 + 12 = b

b = 22

Equation of the line:

`y =(12)/(5)x + 22`