Question

state of the triangles in each pair are similar. If so, say how you know they are similar and complete the similarity statement. Part 5​

Answer 1

Answer: d) similar, SAS similarity, ΔDGH

Explanation:

DG ≡ GC ⇒ G is the midpoint of DC

DH ≡ HB ⇒ H is the midpoint of DB

Therefore, HB is the midsegment of ΔDCB.

By the Midsegment Theorem, HB || BC

By Corresponding Angles Theorem, ∠DGH ≡ ∠DCB

`\underline{Sides}:\\\\(DB)/(DC)= (7)/(14)\rightarrow (1)/(2)\qquad (DH)/(DB)= (7)/(14)\rightarrow (1)/(2)`

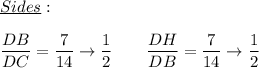
ΔDCB ≅ ΔDGH by Side-Angle-Side Similarity Theorem