Answer:
The inequality that represents the number of cars he needs to sell is:

Explanation:
The commission received by the salesman, can be modeled by the following expression:

Where "x" is the number of sold cars. Since he needs to achieve a commission value of at least 7700, then the inequality that represents the number of cars he needs to sell is:
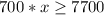
We can solve for "x" in order to find the number of cars he needs to sell:
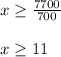
He needs to sell at least 11 cars.