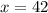Question

Find the value of x in the given figure using properties of parallel lines.

A) 138°
B) 48°
C) Can't be determined.
D) 42°

Answer 1

`x = 42`

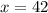
Explanation:

Given

The diagram above

Required

Find x

When two parallel lines are divided in such a way as it is in the above figure;

x and 138 are said to be supplementary angles

The rule about supplementary angles is that, they add up to 180

In other words,

`x + 138 = 180`

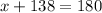
To solve this, we subtract 138 from both sides

`x + 138 - 138= 180 - 138`

`x = 180 - 138`

`x = 42`

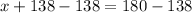
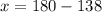
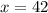
Hence, the value of x when solved using the properties of parallel lines is:
`x = 42`