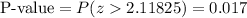Question

A cola manufacturer invited consumers to take a blind taste test. Consumers were asked to decide which of two sodas they preferred. The manufacturer was also interested in what factors played a role in taste preferences. Below is a printout comparing the taste preferences of men and women.

HYPOTHESIS: PROP. X = PROP. YSAMPLES SELECTED FROM soda(brand1,brand2)males (sex=0, males) (NUMBER = 115)females (sex=1, females) (NUMBER = 56)X = malesY = femalesSAMPLE PROPORTION OF X = 0.422018SAMPLE SIZE OF X = 109SAMPLE PROPORTION OF Y = 0.25SAMPLE SIZE OF Y = 52PROPORTION X - PROPORTION Y = 0.172018Z = 2.11825Suppose the manufacturer wanted to test to determine if the males preferred its brand more than the females. Using the test statistic given, for a one-sided test, compute the appropriate p-value for the test.

Answer 1

The P-value for this test is P=0.017.

Explanation:

We have the null and alternative hypothesis:

`H_0: \pi_1-\pi_2=0\\\\H_a:\pi_1-\pi_2> 0`

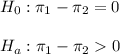
where the subindex 1 refers to the males proportion and the subindex 2 refers to the female proportion.

The difference in proportions is 0.172018 and the test statistic is z=2.11825.

As this is a right-tailed test, the P-value can be calculated using the standard normal distribution table as:

`\text{P-value}=P(z>2.11825)=0.017`